Standard Form to Vertex Form: In geometry, a vertex form or shape is a factor wherein or extra curves, traces, or edges meet. As an effect of this definition, the factor wherein traces meet to shape an attitude and the corners of polygons and polyhedra are vertices. For example, a rectangular has 4 corners, every is referred to as a vertex. The plural shape of the vertex is vertices. The phrase vertex is maximum generally used to indicate the corners of a polygon.
When traces meet at a vertex, they shape a covered attitude. For polygons, the covered attitude at every vertex is an indoors attitude of the polygon. Vertex is likewise occasionally used to suggest the ‘top’ or excessive factor of something, including the vertex of an isosceles triangle, that is the ‘top’ nook contrary to its base, however, this isn’t its strict mathematical definition.
Vertex Form Of Equation
The vertex shape of a parabola’s equation is typically expressed as y = a(x-h)2+ok
- (h, ok) is the vertex
- If a is fine then the parabola opens upwards like a regular “U”.
- If a is negative, then the graph opens downwards like an upside-down “U”.
- If |a| < 1>
- If |a| > 1, the graph of the graph will become narrower (The impact is the alternative of |a| < 1>
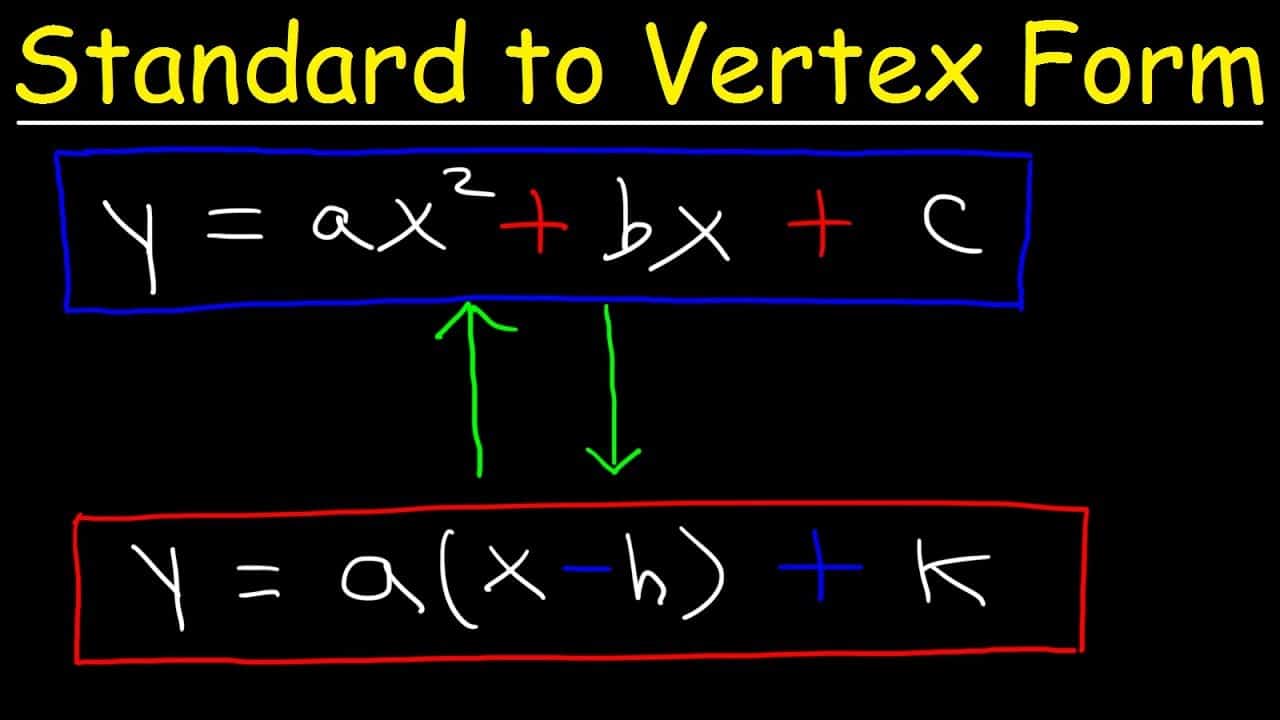
Standard Form To Vertex Form
The popular shape of a quadratic equation is y = ax^2 + bx + c, wherein a, b, and c are coefficients and y and x are variables. It is simpler to remedy a quadratic equation while it’s far in popular shape due to the fact you compute the answer with a, b, and c. However, in case you want to graph a quadratic characteristic or parabola, the system is streamlined while the equation is in vertex shape. The vertex shape of a quadratic equation is y = m(x-h)^2 + ok with m representing the slope of the road and h and ok as any factor on the road.
Factor Coefficient
Factor the coefficient a from the primary phrases of the same old shape equation and area it outdoor of the parentheses. Factoring popular shape quadratic equations includes locating a couple of numbers that upload as much as b and multiply to ac. For instance, in case you are changing 2x^2 – 28x + 10 to vertex shape, you first want to jot down 2(x^2 – 14x) + 10. Suggested – Empirical Formula Definition
Divide Coefficient
Next, divide the coefficient of the x time period in the parentheses with the aid of using. Use the rectangular root assets to then rectangular that quantity. Using that rectangular root assets technique enables to locate the quadratic equation answer with the aid of using taking the rectangular roots of each side. In the example, the coefficient of the x in the parentheses is -14.
Balance Equation
Add the quantity in the parentheses, after which to stability the equation, multiply it with the aid of using the aspect at the outdoor of parentheses and subtract this quantity from the entire quadratic equation. For example, 2(x^2 – 14x) + 10 will become 2(x^2 – 14x + 49) + 10 – 98, considering the fact that 49*2 = 98. Simplify the equation with the aid of using combining the phrases on the end. For example, 2(x^2 – 14x + 49) – 88, considering the fact that 10 – 98 = -88.
Convert Terms
Finally, convert the phrase’s internal parentheses to a squared unit of the shape (x – h)^2. The fee of h is the same as 1/2 of the coefficient of the x time period. For example, 2(x^2 – 14x + 49) – 88 will become 2(x – 7)^2 – 88. The quadratic equation is now in vertex shape. Graphing the parabola in vertex shape calls for the usage of the symmetric residences of the character with the aid of using first selecting a left facet fee and locating the y variable. You can then plot the records factors to graph the parabola. Best Read – What Is Indirect Characterization? Examples